
Table of Contents
ToggleNCERT Solution For Class Class 7, Maths, Lines And Angles, Exercise 5.2 has total six question to practice. Class 7 maths chapter 5 exercise 5.1 is given below. Ex 5.2 class 7 is basically about acute, obtuse and Right angle figure in which students need to find required angles by using suitable method.
NCERT Solution For Class Class 7, Maths, Lines And Angles, Exercise 5.2
Q.1.State the property that is used in each of the following statements?
(i) If a || b, then ∠1 = ∠5.
(ii) If ∠4 = ∠6, then a || b.
(iii) If ∠4 + ∠5 = 180°, then a || b.
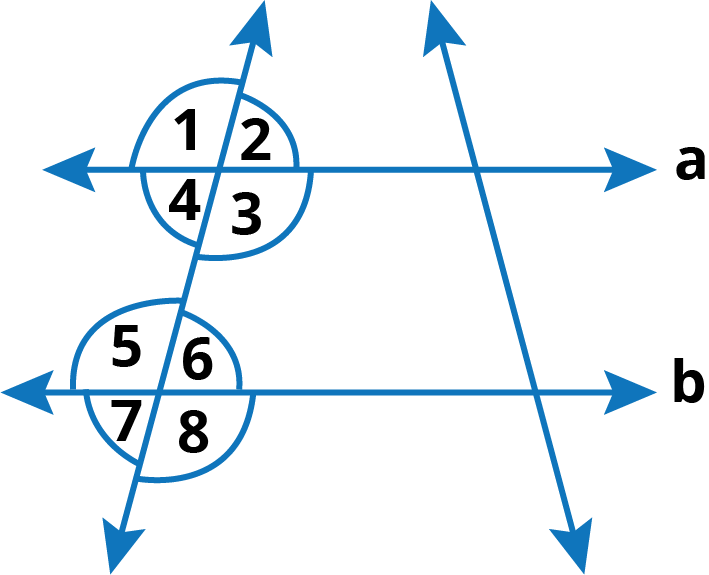
Ans:
(i) If a || b, then ∠1 = ∠5.
Corresponding angles
(ii) If ∠4 = ∠6, then a || b.
Alternate interior angles
(iii) If ∠4 + ∠5 = 180°, then a || b.
Interior angles
Q.2. In the adjoining figure, identify
(i) the pairs of corresponding angles.
(ii) the pairs of alternate interior angles.
(iii) the pairs of interior angles on the same side of the transversal.
(iv) the vertically opposite angles.
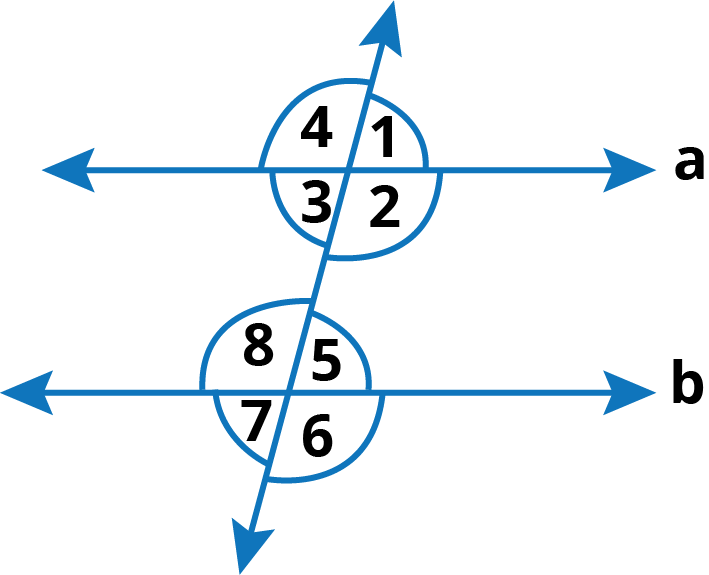
Ans: (i) the pairs of corresponding angles.
The pairs of corresponding angles are, ∠1 and ∠5, ∠4 and ∠8, ∠2 and ∠6, ∠3 and ∠7.
(ii) the pairs of alternate interior angles.
The pairs of alternate interior angle are, ∠2 and ∠8, ∠3 and ∠5.
(iii) the pairs of interior angles on the same side of the transversal.
The pairs of interior angles on the same side of the transversal are ∠2 and ∠5, ∠3 and ∠8.
(iv) the vertically opposite angles.
The vertically opposite angles are, ∠1 and ∠3, ∠5 and ∠7, ∠2 and ∠4, ∠6 and ∠8.
Q.3. In the adjoining figure, p || q. Find the unknown angles.
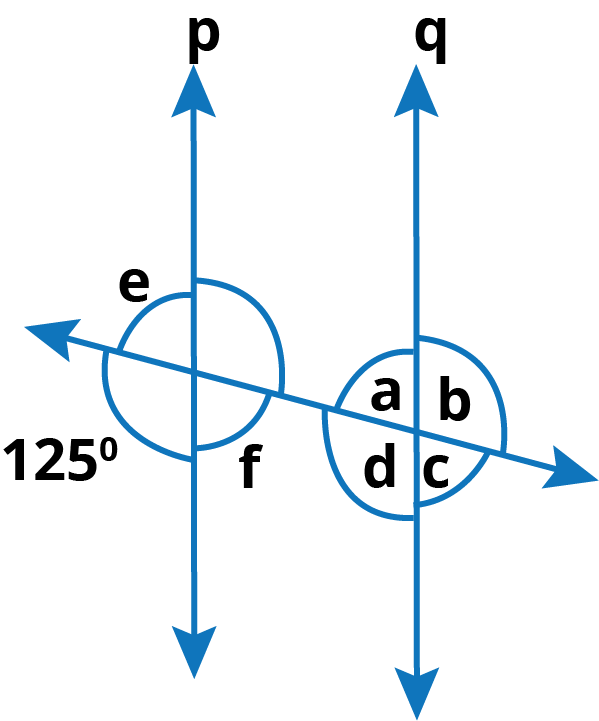
Ans: ∠d = ∠125o [Corresponding angles]
We know that, Linear pair is the sum of adjacent angles is 180o
Then,
⟹ ∠e + 125o = 180o [Linear pair]
⟹ ∠e = 180o – 125o
⟹ ∠e = 55o
Now, ∠e = ∠f = 55o [vertically opposite angles]
∠b = ∠d = 125o
Also, ∠c = ∠f = 55o [Corresponding angles]
∠a = ∠e = 55o
Thus, ∠a = 55o , ∠b = 1250,∠c=550, ∠d = 1250, ∠e = 55o and ∠f = 550
Q.4. Find the value of x in each of the following figures if l || m
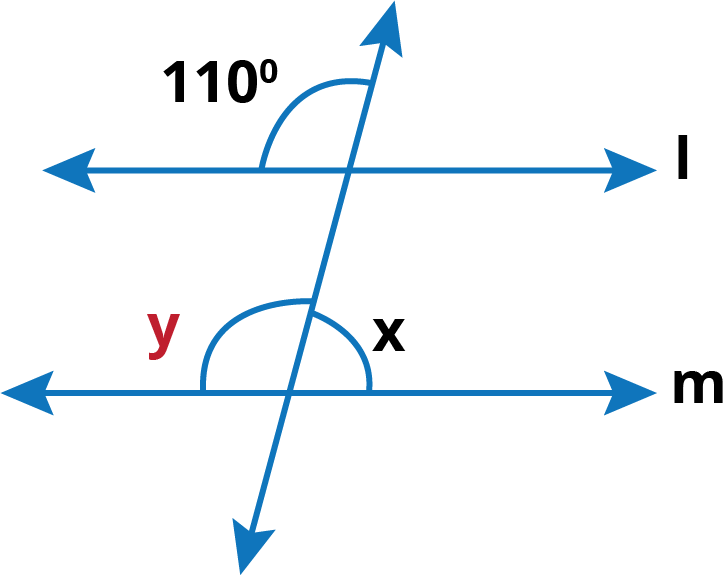

Ans: (i) Let us assume other angle on the line m be ∠y,
Then,
By the property of corresponding angles,
∠y = 110o
We know that Linear pair is the sum of adjacent angles is 180o
Then,
⟹ ∠x + ∠y = 180o
⟹ ∠x + 110o = 180o
⟹ ∠x = 180o – 110o
⟹ ∠x = 70o
(ii) By the property of corresponding angles,
∠x = 100o
Q.5. In the given figure, the arms of two angles are parallel. If ∠ABC = 70º, then find
(i) ∠DGC
(ii) ∠DEF
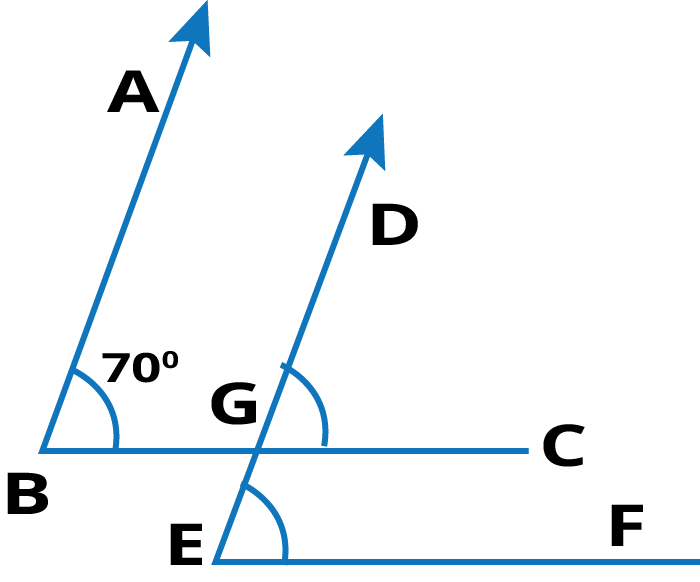
Ans: (i) Given, AB || DG and BC is the transversal line intersecting AB and DG
∠DGC = ∠ABC [Corresponding angles]
Then,
∠DGC = 70o
(ii) Given that BC|| EF and DE is the transversal line intersecting BC and EF
∠DEF = ∠DGC [Corresponding angles]
Then,
∠DEF = 70o
Q.6. In the given figures below, decide whether l is parallel to m.
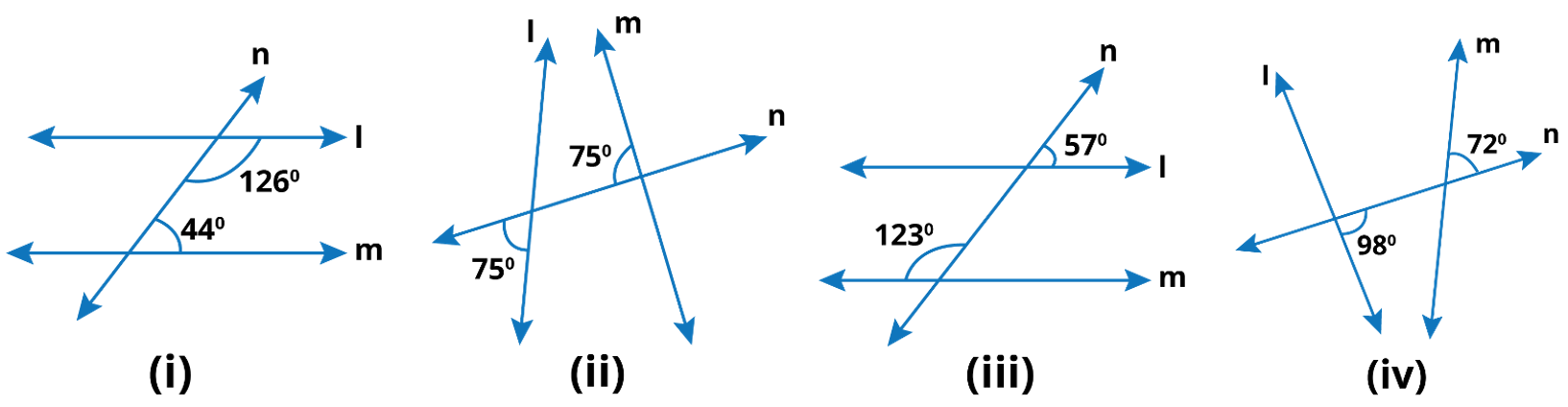
Ans: (i) Let us consider the two lines l and m, n is the transversal line intersecting l and m.
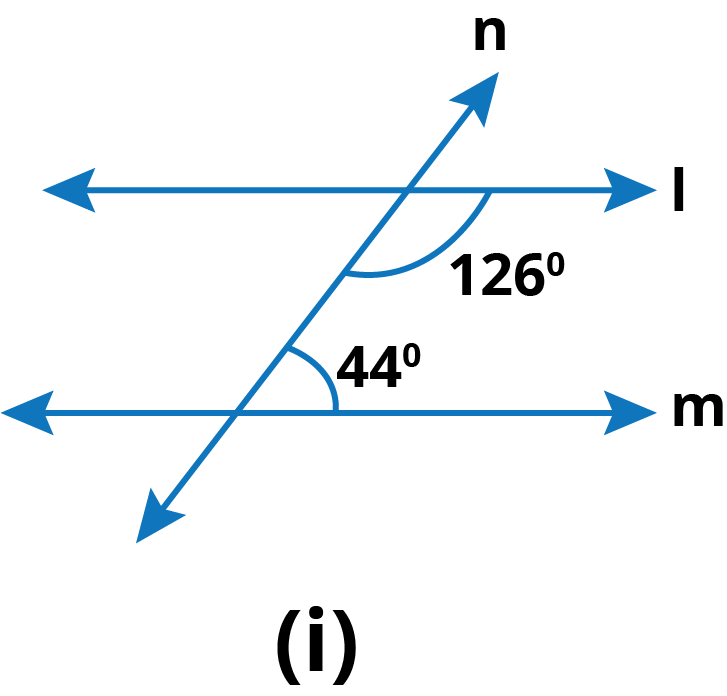
∵ sum of interior angles on the same side of transversal is 180o.
Then,
= 126o + 44o = 170o
But, the sum of interior angles on the same side of transversal is not equal to 180o. So, line l is not parallel to line m.
(ii) Let ∠x be the vertically opposite angle formed due to the intersection of the straight line l and transversal n,

Then, ∠x = 75o
Now, we have two lines l and m, n is the transversal line intersecting l and m.
We know that the sum of interior angles on the same side of transversal is 180o.
Then,
= 75o + 75o
= 150o
But, the sum of interior angles on the same side of transversal is not equal to 180o.
So, line l is not parallel to line m.
(iii) Let ∠x be the vertically opposite angle formed due to the intersection of the Straight-line l and transversal line n,
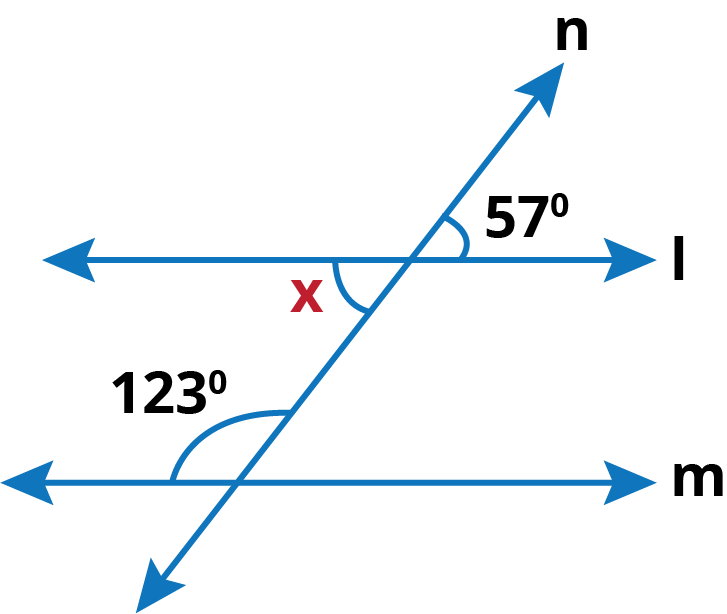
We know that the sum of interior angles on the same side of transversal is 180o.
Then,
= 123o + ∠x
= 123o + 57o
= 180o
∴The sum of interior angles on the same side of transversal is equal to 180o. So, line l is parallel to line m.
(iv) Let us assume ∠x be the angle formed due to the intersection of the Straight line l and transversal line n,
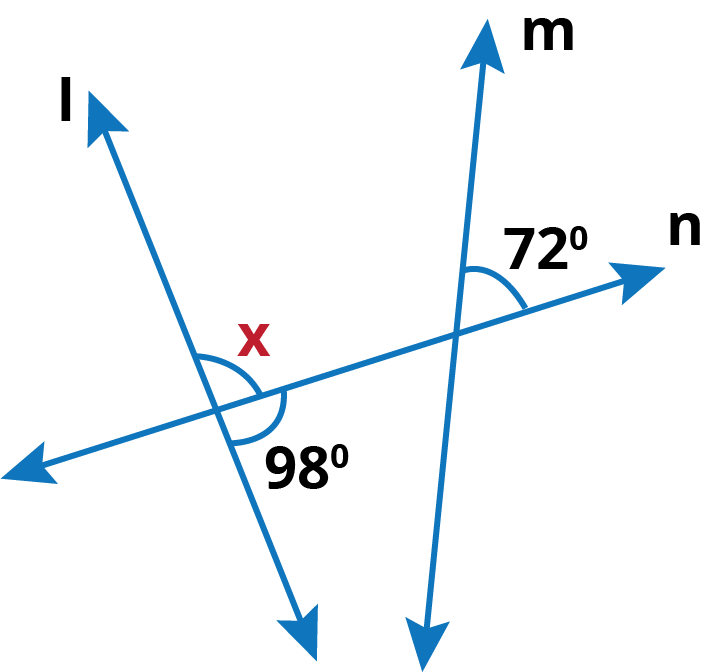
∵ Linear pair is the sum of adjacent angles is equal to 180o.
⟹ ∠x + 98o = 180o
⟹ ∠x = 180o – 98o
⟹ ∠x = 82o
Now, ∠x ≠ 720 [Corresponding angles]
For l and m to be parallel to each other, corresponding angles should be equal. So, Line l is not parallel to line m.

