Class 10, Maths, Chapter 1, Exercise 1.4, Solutions
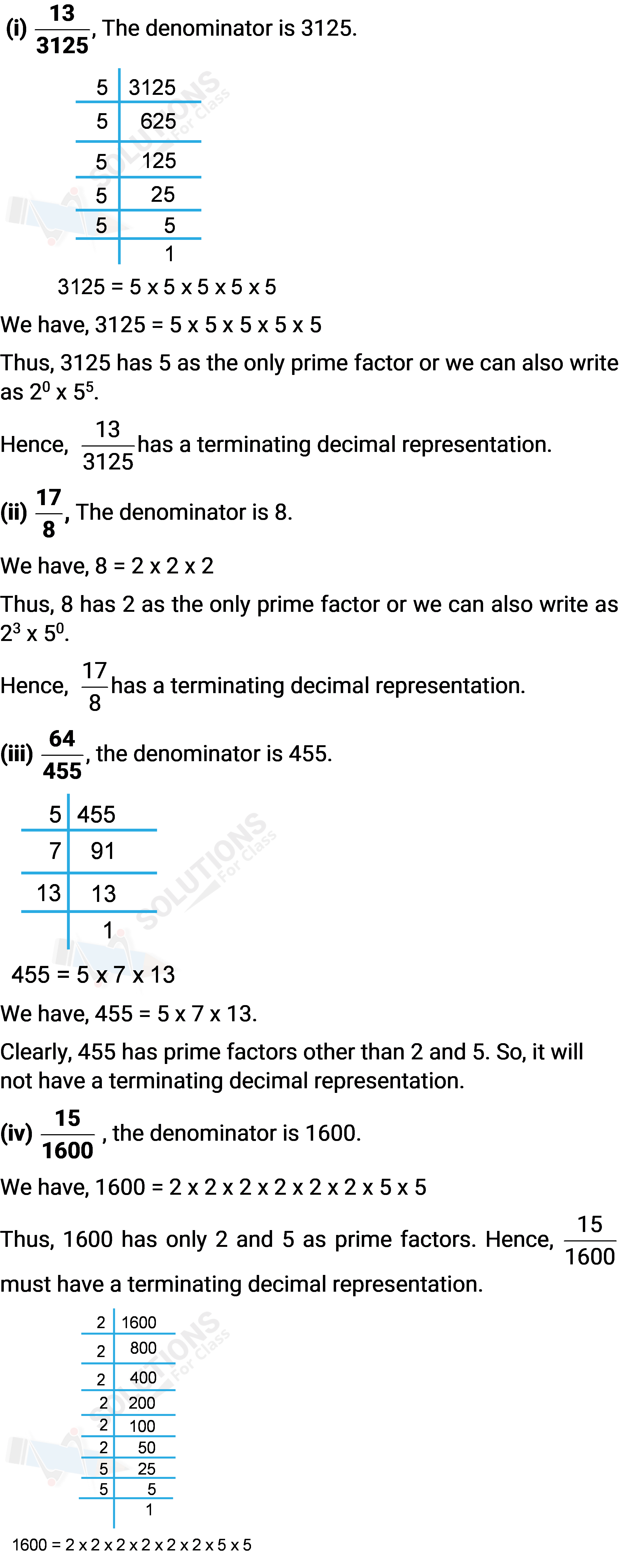
Q.1. Without actually performing the long division, state whether the following rational numbers will have a terminating decimal expansion or a non-terminating repeating decimal expansion:
(i) $\frac{13}{3125}$
(ii) $\frac{17}{8}$
(iii) $\frac{64}{455}$
(iv) $\frac{15}{1600}$
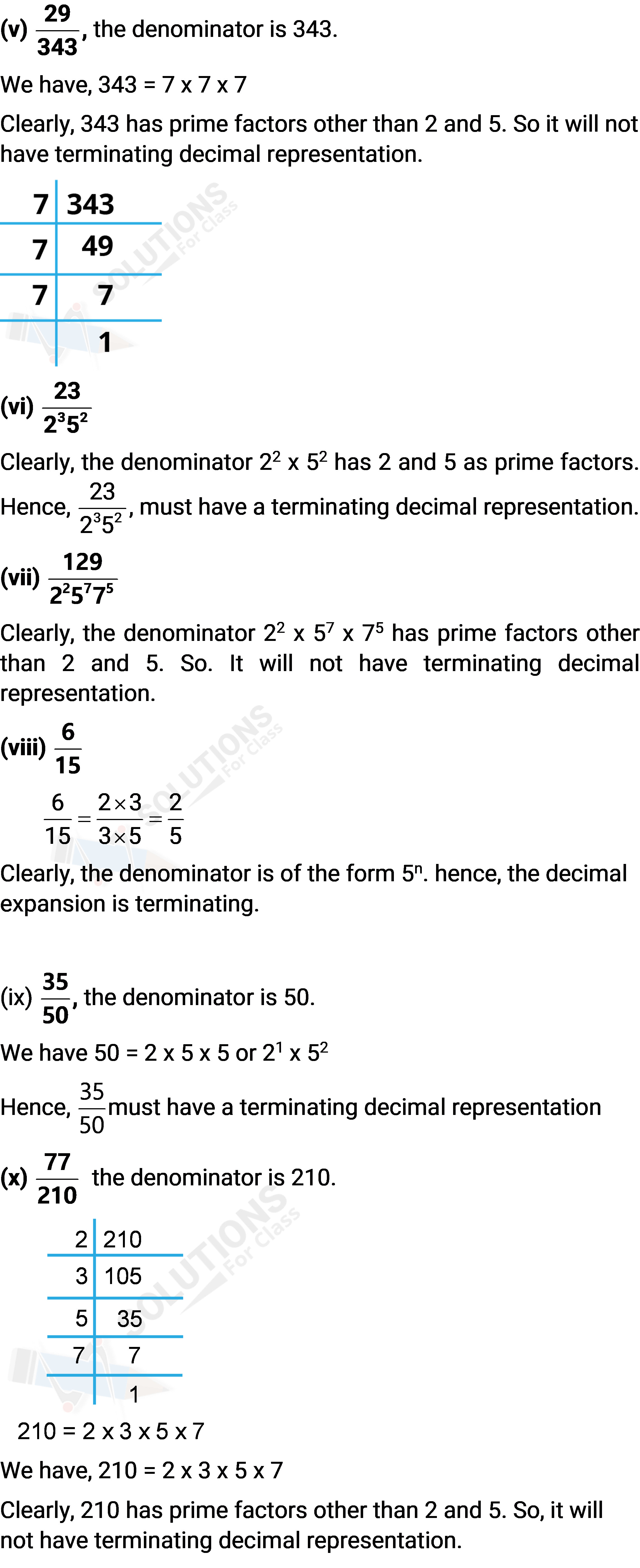
(v) $\frac{29}{343}$
(vi) $\frac{23}{{{2}^{3}}{{5}^{2}}}$
(vii) $\frac{129}{{{2}^{2}}{{5}^{7}}{{7}^{5}}}$
(viii) $\frac{6}{15}$
(ix) $\frac{35}{50}$
(x) $\frac{77}{210}$
Ans: We know that if the denominator of a rational number has no prime factors other than 2 or 5, then it is expressed as a terminating, otherwise it is non- terminating repeating decimal expansion. Thus, we have to check the prime factors of the denominators of each of the given rational numbers.
Q.2. Write down the decimal expansions of those rational numbers in Question 1 above which have terminating decimal expansions.
Ans:
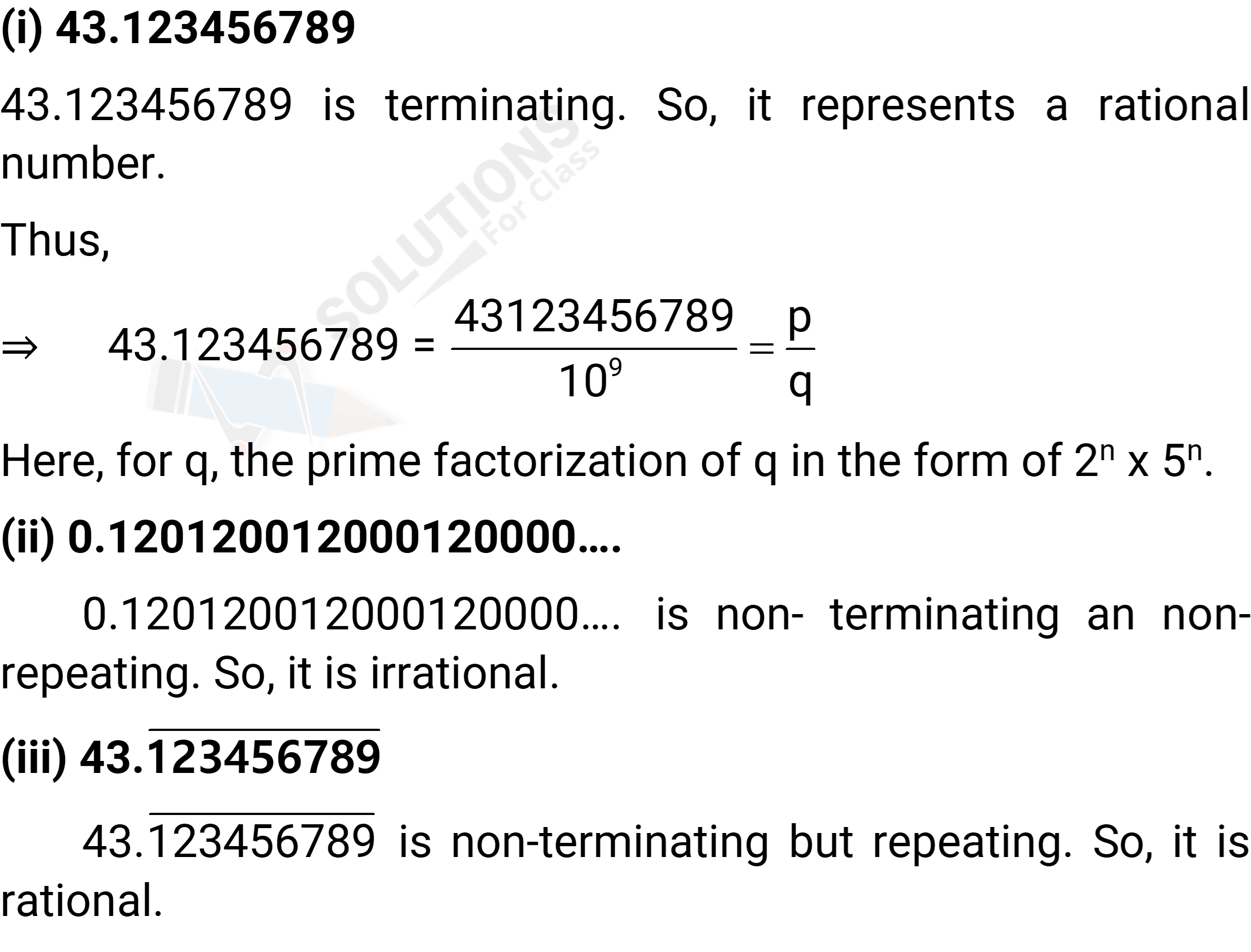
Q.3. The following real numbers have decimal expansions as given below. In each case, decide whether they are rational or not. If they are rational, and of the form , what can you say about the prime factors of q?
(i) 43.123456789
(ii) 0.120120012000120000….
(iii) 43. $\overline{123456789}$
Ans:
