Table of Contents
ToggleClass 7, Maths, Chapter 6, Exercise 6.4 Solutions
Q.1. Is it possible to have a triangle with the following sides?
(i) 2cm,3cm,5cm
(ii) 3 cm, 6 cm, 7 cm
(iii) 6 cm, 3 cm, 2 cm
Ans: Property of triangle: The sum of any two of these numbers is not greater than the third.
(i) 2 cm, 3 cm, 5 cm
⟹ 2 + 3 > 5 No
⟹ 2 + 5 > 3 yes
⟹ 3 + 5 > 2 yes
Hence, the triangle is not possible.
(ii) 3 cm, 6 cm, 7 cm
⟹ 3 + 6 > 7 yes
⟹ 3 + 7 > 6 yes
⟹ 6 + 7 > 3 yes
Hence, the triangle is possible.
(iii) 6 cm, 3 cm, 2 cm
⟹ 6 + 3 > 2 yes
⟹ 6 + 2 > 3 yes
⟹ 3 + 2 > 6 No
Hence, the triangle is not possible.
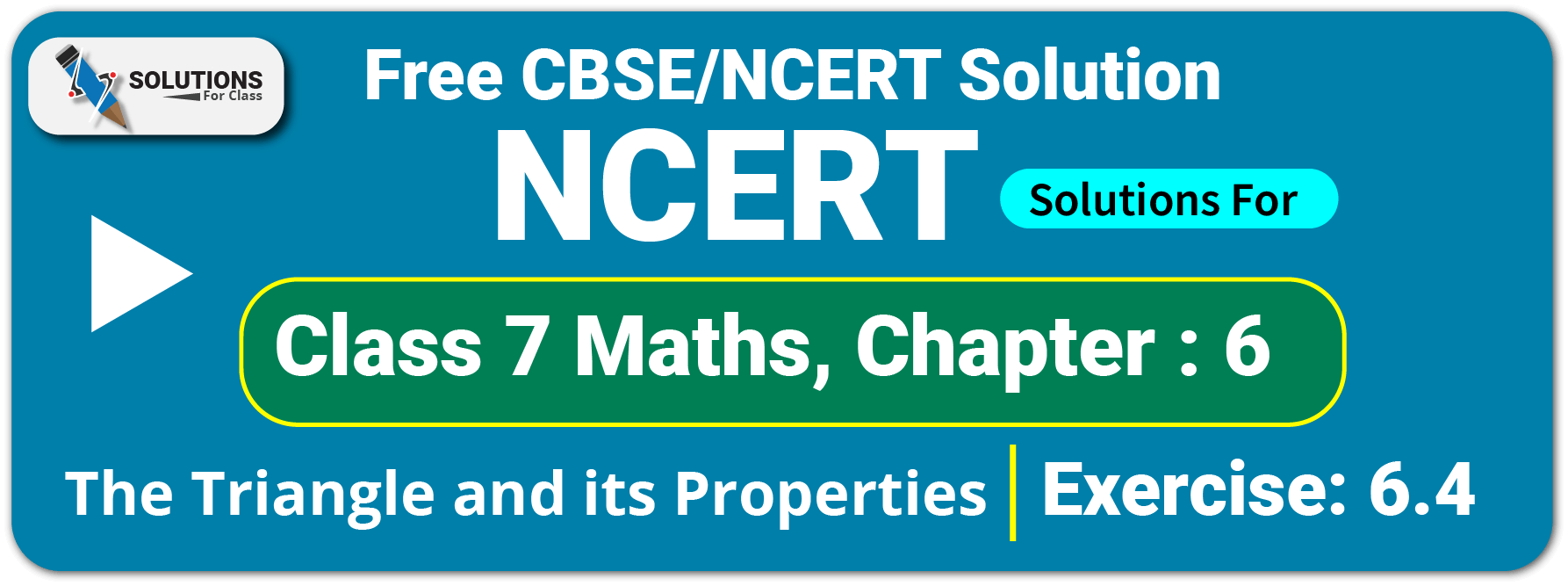
Q.2. Take any point O in the interior of a triangle PQR. Is
(i) OP + OQ > PQ?
(ii) OQ + OR > QR?
(iii) OR + OP > RP?

Ans: If we take any point O in the interior of a triangle PQR and join OR, OP, OQ.
Then, we get three triangles ΔOPQ, ΔOQR and ΔORP .
We know that, the sum of the length of any two sides is always greater than the third side.
(i) Yes, ΔOPQ has sides OP, OQ and PQ. So, OP + OQ > PQ
(ii) Yes, ΔOQR has sides OR, OQ and QR. So, OQ + OR > QR
(iii) Yes, ΔORP has sides OR, OP and PR. So, OR + OP > RP
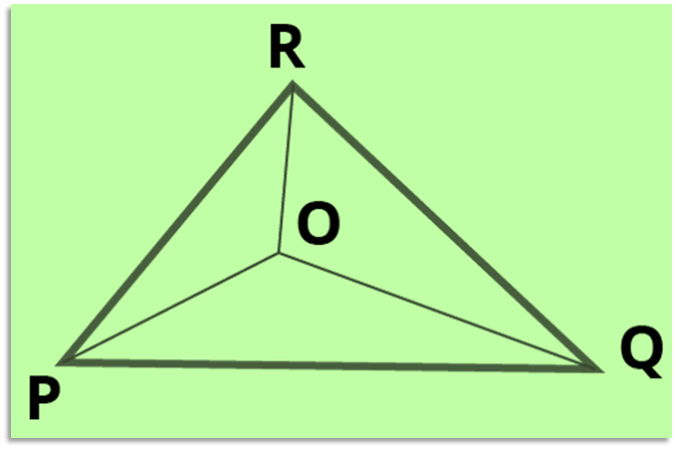
Q.3. AM is a median of a triangle ABC. Is AB + BC + CA > 2 AM?

Ans:
We know that, the sum of the length of any two sides is always greater than the third side.
Therefore, in ΔABM,
AB + BM > AM ……… (i)
Also, in ΔAMC
AC + MC > AM ……… (ii)
By adding equation (i) and (ii) we get,
AB + BM + AC + MC > AM + AM
[in figure, BC = BM + MC]
⟹ AB + AC + (BM + MC) > 2 AM
⟹ AB + BC + AC > 2AM
Hence, the given expression is true.
Q.4. ABCD is a quadrilateral. Is AB + BC + CD + DA > AC + BD?
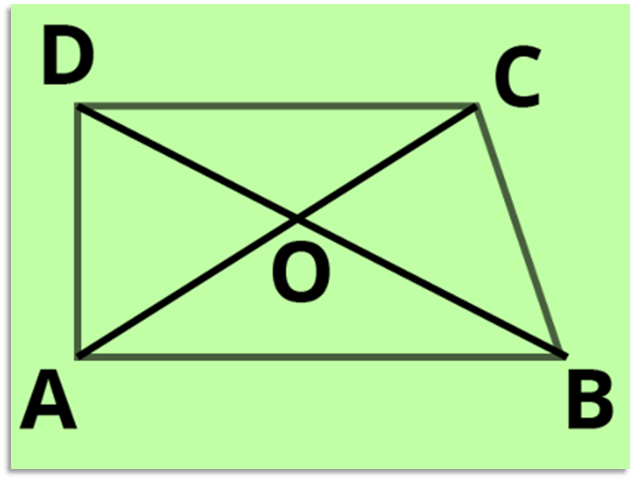
Ans: We know that, the sum of the length of any two sides is always greater than the third side.
In Δ ABC, AB + BC > AC …………… (i)
In Δ ADC, AD + DC > AC …………… (ii)
In Δ DCB, DC + CB > DB …………… (iii)
In Δ ADB, AD + AB > DB …………… (iv)
Adding equations (i), (ii), (iii) and (iv), we get
∴ AB + BC + AD + DC + DC + CB + AD + AB > AC + AC + DB + DB
⟹ (AB + AB) + (BC + BC) + (AD + AD) + (DC + DC) > 2AC + 2DB
⟹ 2AB + 2BC + 2AD + 2DC > 2(AC + DB)
Take out 2 on both the side,
⟹ 2(AB + BC + AD + DC) > 2(AC + DB)
⟹ AB + BC + AD + DC > AC + DB
⟹ AB + BC + CD + DA > AC + DB
Hence, the given expression is true.
Q.5. ABCD is quadrilateral. Is AB + BC + CD + DA < 2 (AC + BD)?

Ans: We know that, the sum of the length of any two sides is always greater than the third side.
In Δ AOB, AB < OA + OB …………… (i)
In Δ BOC, BC < OB + OC …………… (ii)
In Δ COD, CD < OC + OD …………… (iii)
In Δ AOD, DA < OD + OA …………… (iv)
Adding equations (i), (ii), (iii) and (iv), we get
⟹ AB + BC + CD + DA < OA + OB + OB + OC + OC + OD + OD + OA
⟹ AB + BC + CD + DA < 2OA + 2OB + 2OC + 2OD
⟹ AB + BC + CD + DA < 2[(AO + OC) + (DO + OB)]
In figure, AC = AO + OC and BD = DO + OB.
⟹ AB + BC + CD + DA < 2(AC + BD)
Q.6. The lengths of two sides of a triangle are 12 cm and 15 cm. Between what two measures should the length of the third side fall?
Ans: We know that, the sum of the length of any two sides is always greater than the third side.
According to question, it is given that two sides of triangle are 12 cm and 15 cm.
So, the third side length should be less than the sum of other two sides,
∴ 12 + 15 = 27 cm.
Then, it is given that the third side is cannot not be less than the difference of the two sides, 15 – 12 = 3 cm
Hence,, the length of the third side falls between 3 cm and 27 cm.
NCERT Solutions For Class 7 Maths, Chapter 6, The Triangle and its Properties (All Exercises)
Class 7, Maths, Chapter 6, The Triangle and Its Properties
Class 7, Maths, Chapter 6, The Triangle and Its Properties, Exercise 6.1
Class 7, Maths, Chapter 6, The Triangle and Its Properties, Exercise 6.2
Class 7, Maths, Chapter 6, The Triangle and Its Properties, Exercise 6.3
Class 7, Maths, Chapter 6, The Triangle and Its Properties, Exercise 6.4 ← You are here
Class 7, Maths, Chapter 6, The Triangle and Its Properties, Exercise 6.5

